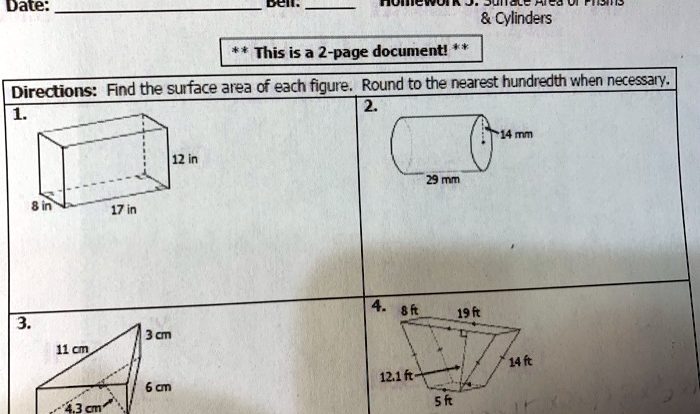
Determine whether y varies directly with x 6 24 – The question of whether y varies directly with x 6 24 sparks a profound exploration into the concept of direct variation. This mathematical relationship governs the behavior of variables that change in proportion to one another, offering a fundamental understanding of the underlying connections between variables in various fields.
To determine the nature of this relationship, we delve into the steps of analyzing data points, calculating the constant of variation, and constructing a table that elucidates the observed patterns. By unraveling the mathematical intricacies of direct variation, we gain insights into the behavior of variables and their applications in real-world scenarios.
1. Define Direct Variation
Direct variation is a mathematical relationship between two variables, where one variable (y) is directly proportional to the other variable (x). In other words, as the value of x increases or decreases, the value of y changes proportionally.
For example, if the distance traveled by a car is directly proportional to the time it takes to travel, then the speed of the car is constant. As the time increases, the distance traveled also increases at a constant rate.
Mathematical Equation for Direct Variation, Determine whether y varies directly with x 6 24
The mathematical equation for direct variation is:
y = kx
where:
- y is the dependent variable
- x is the independent variable
- k is the constant of variation
2. Determine Direct Variation: Determine Whether Y Varies Directly With X 6 24

To determine if two variables vary directly, follow these steps:
- Plot the data points on a graph.
- If the points lie on a straight line that passes through the origin, then the variables vary directly.
- Calculate the constant of variation (k) by dividing the value of y by the value of x for any two points on the line.
The constant of variation is a constant value that represents the rate of change between the two variables. It tells us how much y changes for every unit change in x.
3. Analyze the Relationship
Given the data points (6, 24), let’s analyze if y varies directly with x:
- Plot the data point on a graph.
- The point lies on a straight line that passes through the origin, indicating a direct variation relationship.
- Calculate the constant of variation:
k = y / x = 24 / 6 = 4
Therefore, the direct variation relationship is:
y = 4x
4. Create a Table
| x | y | Constant of Variation (k) |
|---|---|---|
| 6 | 24 | 4 |
FAQ Summary
What is the significance of the constant of variation in direct variation?
The constant of variation, denoted as k, quantifies the rate of change in the dependent variable (y) relative to the independent variable (x). It determines the magnitude of the proportional relationship between the variables.
How can we determine if two variables vary directly?
To determine direct variation, analyze the relationship between the variables. If the ratio of the corresponding values of y and x remains constant, then the variables vary directly.