Mean median mode range worksheet answer key – Embark on a statistical adventure with our comprehensive guide to mean, median, mode, and range, unveiling the concepts, calculations, and applications of these fundamental statistical measures. Dive into a world of data analysis, unlocking the secrets to describe and compare datasets with precision.
In this captivating exploration, we will delve into the definitions and concepts of mean, median, mode, and range, providing real-world examples to illuminate their practical significance. Step-by-step instructions will empower you to calculate these measures with confidence, equipping you with the tools to analyze data effectively.
Mean, Median, Mode, and Range: Mean Median Mode Range Worksheet Answer Key
Mean, median, mode, and range are four measures of central tendency that are used to describe a set of data. Each measure has its own strengths and weaknesses, and it is important to understand when to use each one.
Definitions and Concepts
- Meanis the average of a set of numbers. To calculate the mean, add up all the numbers in the set and then divide by the number of numbers.
- Medianis the middle number in a set of numbers. To calculate the median, first put the numbers in order from least to greatest. If there is an even number of numbers, the median is the average of the two middle numbers.
- Modeis the number that occurs most often in a set of numbers. A set of numbers can have more than one mode.
- Rangeis the difference between the largest and smallest numbers in a set of numbers.
Calculating Mean, Median, Mode, and Range
Here are the steps for calculating mean, median, mode, and range:
- Mean:Add up all the numbers in the set and then divide by the number of numbers.
- Median:Put the numbers in order from least to greatest. If there is an even number of numbers, the median is the average of the two middle numbers.
- Mode:Find the number that occurs most often in the set of numbers.
- Range:Subtract the smallest number in the set from the largest number in the set.
Comparing Mean, Median, Mode, and Range
Each measure of central tendency has its own strengths and weaknesses. The mean is a good measure of the average value of a set of numbers, but it can be skewed by outliers. The median is not as sensitive to outliers as the mean, but it can be difficult to calculate for large sets of data.
The mode is the easiest measure of central tendency to calculate, but it can be misleading if there is more than one mode.
The range is a good measure of the spread of a set of numbers, but it can be difficult to interpret for large sets of data.
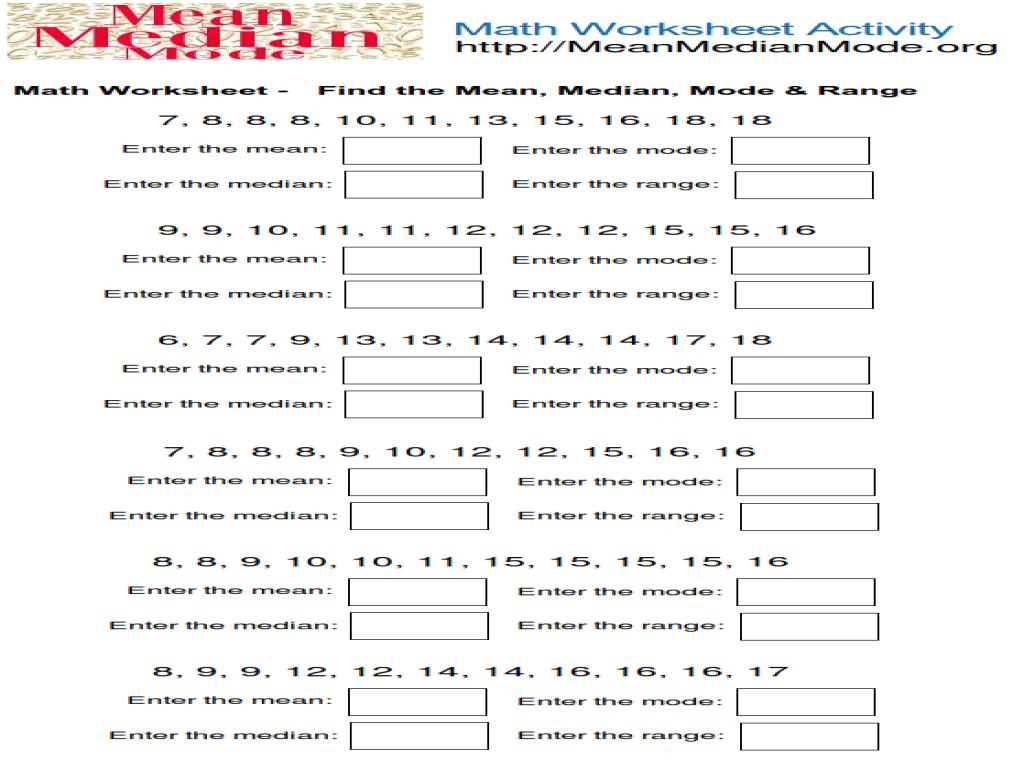
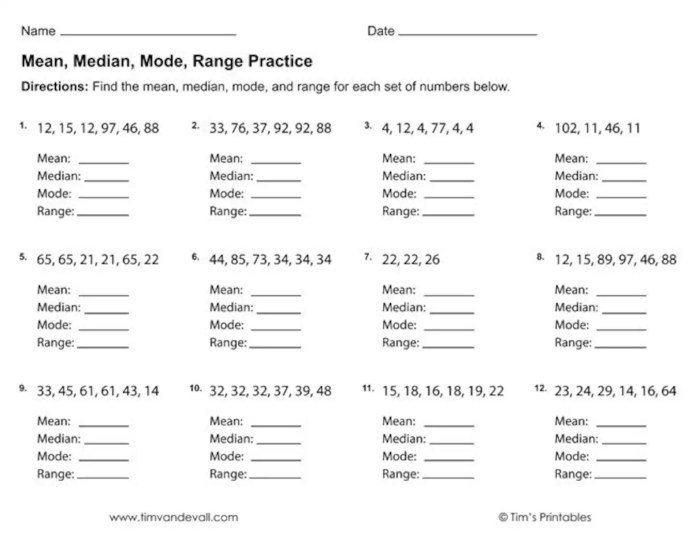
Worksheet and Answer Key, Mean median mode range worksheet answer key
Here is a worksheet with practice problems for calculating mean, median, mode, and range.
| Problem | Answer |
|---|---|
| Find the mean, median, mode, and range of the following set of numbers: 1, 3, 5, 7, 9 | Mean: 5, Median: 5, Mode: 1, 3, 5, 7, 9, Range: 8 |
| Find the mean, median, mode, and range of the following set of numbers: 2, 4, 6, 8, 10 | Mean: 6, Median: 6, Mode: 2, 4, 6, 8, 10, Range: 8 |
| Find the mean, median, mode, and range of the following set of numbers: 1, 2, 3, 4, 5, 6, 7 | Mean: 4, Median: 4, Mode: 1, 2, 3, 4, 5, 6, 7, Range: 6 |
Applications of Mean, Median, Mode, and Range
Mean, median, mode, and range are used in a variety of applications, including:
- Statistics:Mean, median, mode, and range are used to describe the central tendency and spread of a set of data.
- Finance:Mean, median, mode, and range are used to analyze financial data, such as stock prices and interest rates.
- Engineering:Mean, median, mode, and range are used to analyze engineering data, such as the strength of materials and the efficiency of machines.
- Science:Mean, median, mode, and range are used to analyze scientific data, such as the results of experiments and the measurements of physical properties.
Detailed FAQs
What is the difference between mean, median, and mode?
Mean is the average of a dataset, median is the middle value, and mode is the most frequently occurring value.
How do I calculate the range of a dataset?
Subtract the smallest value from the largest value in the dataset.
When should I use mean instead of median?
Mean is more appropriate when the data is normally distributed, while median is more robust to outliers.